Higher Level Geometry and Trigonometry

Radians

• Although degrees are the units you are familiar with, the IB uses radians to communicate angles
• They relate the size of an angle to the distance to a point round the circumference of a circle
• 360° = 2π radians

Length of an arc

• Formula (given in formula booklet): length = radius x θ (in radians)

Area of a sector

• Formula (given in formula booklet): Area= 0.5 x θ (in radians) x radius x radius
• So basically: 0.5 x angle x radius squared

Sine rule implication

• When using the sine rule to find angles, there are two possible answers
• To find both, use your first answer, and do (180 – Answer) to find the other possible answer

Tangent function

• It is defined as the ratio of the sine function to the cosine function
• tan θ = sin θ ÷ cos θ
• This formula is included in the formula booklet

Pythagorean identity

• Straight forward formula to find cos or sin of θ
• Simply use roots and rearranging to find the value of the desired function
• This formula is given in the formula booklet:
  

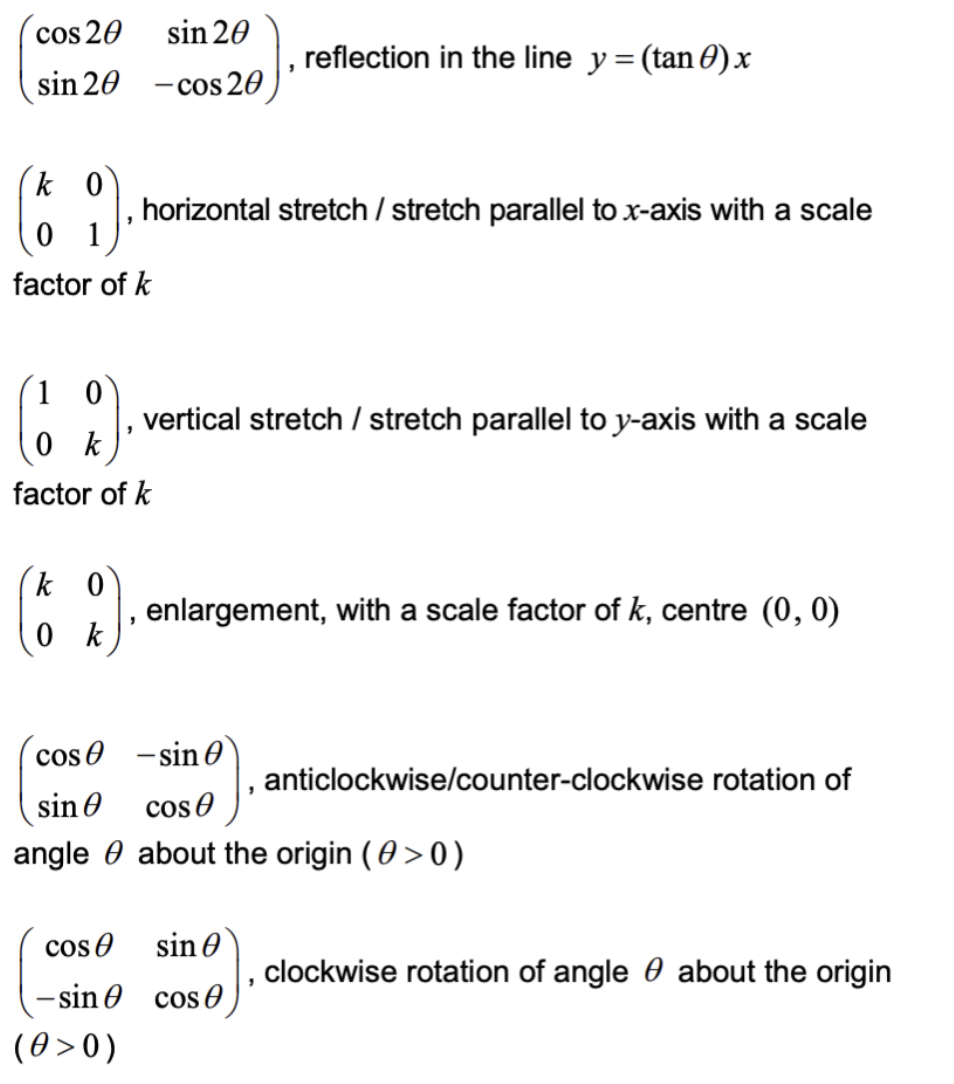
Matrice transformations

• These functions are given in the formula booklet
• They are used to transform matrices
• To check if they are correct, use your GDC to plot the position of each point
• Formulas:
  

Combining matrix transformations

• If the transformation with matrix A is followed by the transformation with matrix B, the combined transformation has matrix BA